Spring Oscillator#
A wooden train is attached to two linear springs of spring constant \(k\) in a closed box. The train is displaced from the center of the box by \(x_0 \ \rm{m}\).
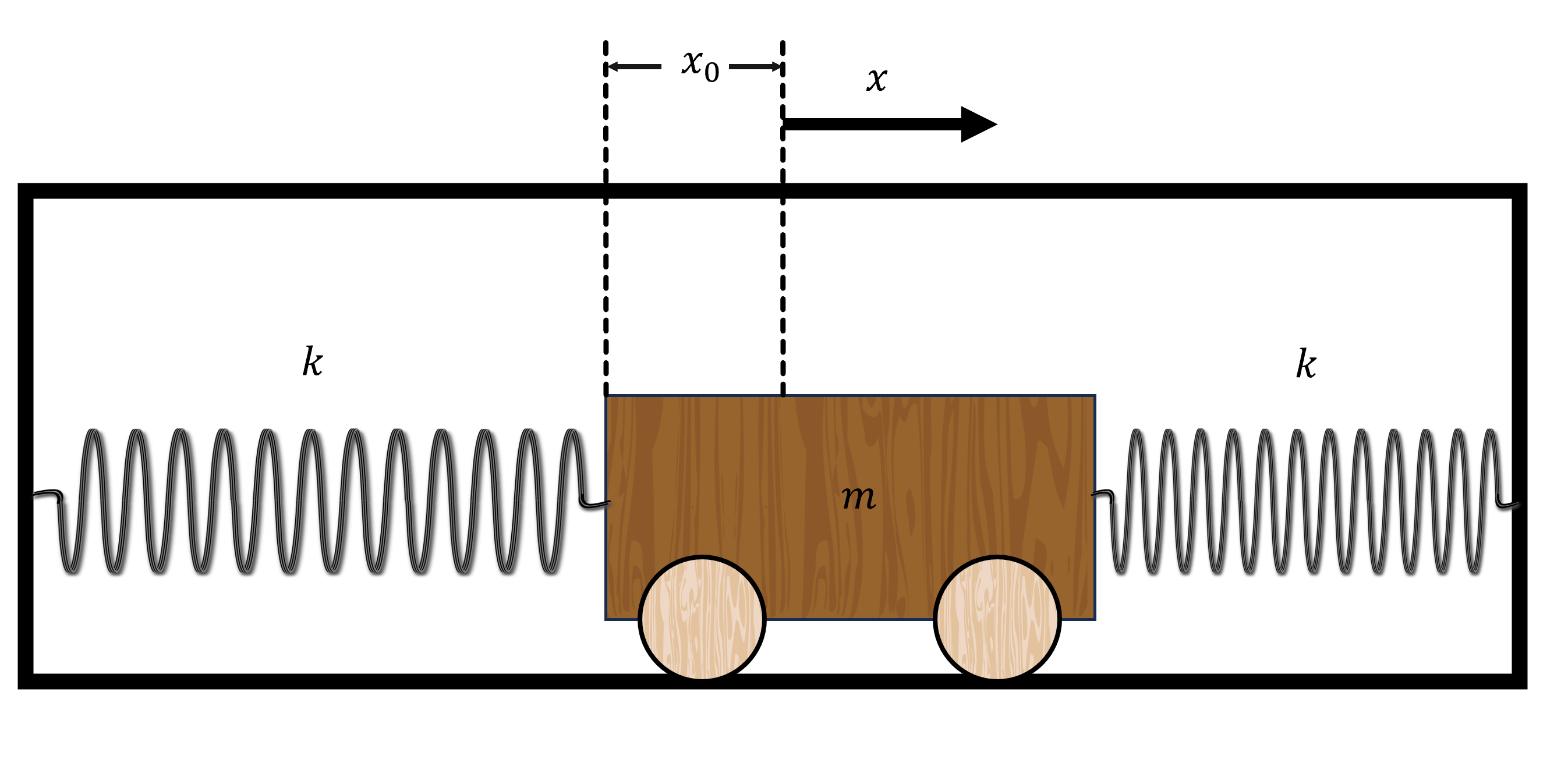
Part 1#
If the train is released from rest and it moves along the frictionless floor, what is the total force in the x-direction acting on the train at the point of release?
\(m= {{ params.m }} \ \rm{kg}\), \(x\_{0}= {{ params.x_0 }} \ \rm{cm}\), \(k = {{ params.k }} \ \rm{N/m}\).
Answer Section#
Please enter in a numeric value in \(N\).
Part 2#
What is the maximum acceleration of the train?
Answer Section#
Please enter in a numeric value in \(ms^{-2}\).
Part 3#
By obtaining the displacement of the train as a function of time, determine the period of oscillation of the train i.e the time that it takes for the train to return back to its starting position.
Answer Section#
Please enter in a numeric value in \(s\).
Part 4#
If the spring stiffness constant is quadrupled, what is the new period of oscillation?
Answer Section#
Please enter in a numeric value in \(s\).
Attribution#
Problem is licensed under the CC-BY-NC-SA 4.0 license.

